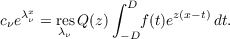|
If f ∈ L(−D,D) and Q(z) is a
meromorphic function whose poles, all simple, forms a sub-set of the set
{λν}(ν = 0,±1,±2,⋯), then the C.E.S. (Cauchy exponential series) of f with respect
to Q(z) is ∑
cνeλνx, where
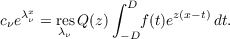
Suppose we are given a class A of functions f each of which can be ‘represented’
in (−D,D) by its C.E.S. with respect to Q(z). We define a set of neighbourhoods U
of {λν}. Then {λν} is stable if there is a U such that to each {κν}∈ U there
corresponds a meromorphic function q(z) whose poles, all simple, form a sub-set of
{κν} and which is such that each f ∈ A can be represented in (−D,D)
by its C.E.S. with respect to q(z); and {λν} is unstable if there is no such
neighbourhood.
The case in which λν = iν, A is BV [−D,D], ‘representation of f in (−D,D)’
means ‘∑
|ν|≦ncνeλνx → 1∕2(f(x+) + f(x−)) boundedly within (D,D)’ is
considered. It is shown, in particular, that with reasonable conditions on
the set of neighbourhoods U, {iν} is unstable if D > 1∕2π, and stable if
D = 1∕2π.
|