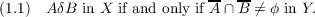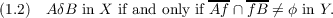|
In the theory of proximity
spaces of Efremovic, (The geometry of proximity, Mat. Sbornic, N.S. 31 (73), (1952),
189–200) the result: A set X with a binary relation “A close to B” is a proximity
space if and only if there exists a compact Hausdorff space Y in which X can be
imbedded so that A is close to B in X if and only if A meets B in Y (A denotes the
closure of the set A) (Y. M. Smirnov, on proximity spaces, Mat. Sbornic, N.S. 31
(73), (1952), 543–574.) Raises the question: Can we display a set of axioms for a
binary relation δ on the power set of a set X so that the system (X,δ) satisfies these
axioms if and only if there is a topological space Y in which X can be imbedded so
that
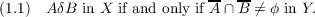
In (M. W. Lodato, On topologically induced generalized proximity relations,
Proc. Amer. Math. Soc. vol. 15, no. 3, June 1964, pp. 417–422), it is shown that
an affirmative answer can be given if Y is T1 and if X is regularly dense in Y . The
clusters of S. Leader, On clusters in proximity spaces, Fund. Math. 47 (1959),
205–213, were used in (M. W. Lodato, On topologically induced generalized
proximity relations, Proc. Amer. Math. Soc. vol. 15, no. 3, June 1964,
pp. 417–422). The present paper generalized this notion and thus relaxes the
condition that X be regularly dense in Y . We actually characterize every system
(X,δ) for which there exists a mapping f (not necessarily one-to-one) of X into a
Hausdorff space Y such that
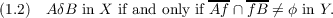
|