|
If a string has a density given
by a nonnegative integrable function ρ defined on the interval [0,a] and is
fixed at its end points under unit tension, then the natural frequencies of
vibration of the string are determined by the eigenvalues of the differential
system
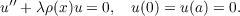 | (1) |
As is well known, the eigenvalues of (1) form a positive strictly increasing sequence of
numbers which depend on the density ρ(x). We denote them accordingly
by
![0 < λ1[ρ] < λ2[ρ] < ⋅⋅⋅ < λn[ρ] < ⋅⋅⋅ .](a011x.png)
In this paper we find lower bounds for these eigenvalues when the density ρ
satisfies a Lipschitz condition with Lipschitz constant H and ∫
0aρdx = M. The
bounds will be in terms of M and H.
|





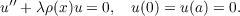
![0 < λ1[ρ] < λ2[ρ] < ⋅⋅⋅ < λn[ρ] < ⋅⋅⋅ .](a011x.png)
