Isomorphism invariants for Abelian groups modulo bounded groups

Ronald J. Ensey

Vol. 24 (1968), No. 1, 71–91

DOI: 10.2140/pjm.1968.24.71

Abstract

Let 𝒜 be the category of Abelian groups, let ℬ be the class of bounded Abelian groups, and form the quotient category 𝒜∕ℬ. The principal goal of this paper is a complete set of invariants for direct sums of countable reduced p-groups, such groups considered as objects of the category 𝒜∕ℬ. Specifically, it will be shown that two direct sums of countable reduced p-groups G and H are isomorphic in 𝒜∕ℬ if and only if there is an integer k ≧ 0 such that for all ordinal numbers α and all integers r ≧ 0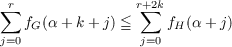

and

where f_G(β) and f_1J(β) denote the β-th Ulm invariants of G and H, respectively. Thus a complete set of 𝒜∕ℬ-isomorphism invariants for such groups is an equivalence class of Ulm invariants, the equivalence relation being given by these two inequalities.

Mathematical Subject Classification

Primary: 20.30

Milestones

Received: 13 December 1966

Published: 1 January 1968