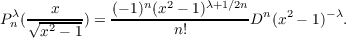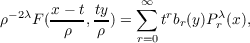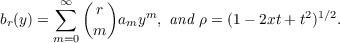Vol. 29, No. 1, 1969
Download this article
Download this article. For screen
For printing
Recent Issues
Vol. 332: 1  2
Vol. 331: 1  2
Vol. 330: 1  2
Vol. 329: 1  2
Vol. 328: 1  2
Vol. 327: 1  2
Vol. 326: 1  2
Vol. 325: 1  2
Online Archive
Volume:
Issue:
     
The Journal
About the journal
Ethics and policies
Peer-review process
 
Submission guidelines
Submission form
Editorial board
Officers
 
Subscriptions
 
ISSN 1945-5844 (electronic)
ISSN 0030-8730 (print)
 
Special Issues
Author index
To appear
 
Other MSP journals
A bilateral generating function for the ultraspherical polynomials

S. K. Chatterjea
Vol. 29 (1969), No. 1, 73–76
DOI: 10.2140/pjm.1969.29.73
Abstract

The following differentiation formula for the Ultraspherical polynomials Pnλ(x) was given by Tricomi:

λ ---x--- (− 1)n(x2 −-1)λ+1∕2n n 2 −λ Pn(√x2-−-1) = n! D (x − 1) .
(1.1)

The object of this paper is to point out that the formula of Tricomi leads us to the following bilateral generating function for the Ultraspherical polynomials:

Theorem. If F(x,t) = ∑ m=0∞amtmPmλ(x), then

x − t ty ∑∞ ρ−2λF(-----,--) = trbr(y)Pλr (x), ρ ρ r=0
(1.2)

where

∞ ( ) br(y) = ∑ r amym, and ρ = (1 − 2xt+ t2)1∕2. m=0 m

Starting from the formula (1.2), one can derive a large number of bilateral generating functions for the Ultraspherical polynomials by attributing different values to am.
Mathematical Subject Classification
Primary: 33.40
Milestones
Received: 20 April 1968
Published: 1 April 1969
Authors
S. K. Chatterjea