Local regularity of solutions of Sobolev-Galpern partial differential equations

Ralph Edwin Showalter

Vol. 34 (1970), No. 3, 781–787

DOI: 10.2140/pjm.1970.34.781

Abstract

Let M and L be elliptic differential operators of orders 2m and 2l, respectively, with m ≦ l The existence and uniqueness of a solution to the abstract mixed initial and boundary value problem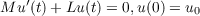

was established for u₀ given in the domain of the infinitesimal generator of a strongly-continuous semi-group. The purpose of this paper is to show that this semi-group is holomorphic and then obtain differentiability results for the solution and convergence of this solution to the initial function u₀ as t ↓ 0.

Mathematical Subject Classification

Primary: 35.24

Milestones

Received: 23 October 1969

Published: 1 September 1970