|
|||||

|

|
|

|

|
|
On the univalence of
some analytic functions
Ghulam M. Shah |
|
Vol. 43 (1972), No. 1, 239–250
|
Mathematical Subject Classification
Primary: 30A32
|
Milestones
Received: 1 July 1971
Revised: 28 January 1972
Published: 1 October 1972
|
Authors |
| Ghulam M. Shah | |
 |
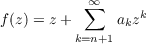
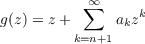
![Re(f(z)∕[λf(z)+ (1− λ)g(z)]) > 0](a222x.png)
![|f(z)∕[λf(z)+ (1− λ)g(z)]− 1| < 1 for |z| < 1,0 ≦ λ < 1.](a223x.png)
