On the differentiability of multifunctions

F. S. De Blasi

Vol. 66 (1976), No. 1, 67–81

DOI: 10.2140/pjm.1976.66.67

Abstract

A new concept of differential for a multifunction is introduced. Here by a multifunction we mean a map from a Banach space X to some specified family of non void subsets of a Banach space Y . The comparison with another definition due to Lasota and Strauss shows that if a multifunction admits both differentials, these must be equal. The results are applicable to the perturbation theory for multivalued differential equations in a Banach space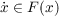

in a neighborhood of a rest position.

Mathematical Subject Classification 2000

Primary: 58C20

Secondary: 46G05, 34G05

Milestones

Received: 17 July 1975

Revised: 12 February 1976

Published: 1 September 1976