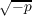Symmetries for sums of the Legendre symbol

Wells Johnson and Kevin J. Mitchell

Vol. 69 (1977), No. 1, 117–124

DOI: 10.2140/pjm.1977.69.117

Abstract

Symmetries are presented for sums of the Legendre symbol (a∕p) over certain subintervals of (0,p). The results follow from an elementary theorem which establishes linear relations among these sums. The list of subintervals of (0,p) for which the number of quadratic residues equals the number of non-residues is extended. Some simple applications to the determination of the class number of the imaginary. quadratic fields Q() are also given.

Mathematical Subject Classification

Primary: 10A20, 10A20

Milestones

Received: 5 August 1976

Published: 1 March 1977