Minimal and maximal solutions of nonlinear boundary value problems

Recent Issues

Vol. 332: 1 2

Vol. 331: 1 2

Vol. 330: 1 2

Vol. 329: 1 2

Vol. 328: 1 2

Vol. 327: 1 2

Vol. 326: 1 2

Vol. 325: 1 2

Online Archive

Volume:

Issue:

The Journal

ISSN 1945-5844 (electronic)

ISSN 0030-8730 (print)

Vol. 71 (1977), No. 1, 13–20

DOI: 10.2140/pjm.1977.71.13

This paper is concerned with the construction of the minimal and the maximal solutions of the nonlinear boundary value problem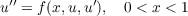

u′′ = f (x,u,u′), 0 < x < 1

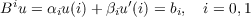Biu = αiu(i)+ βiu′(i) = bi, i = 0,1

under rather mild assumptions on f. In particular, no assumption of monotonicity is made on f(x,u,u′) either in u or u′.

Primary: 34B15

Received: 14 September 1975

Published: 1 July 1977