Approximation by rational modules on nowhere dense sets

James Li-Ming Wang

Vol. 80 (1979), No. 1, 293–295

DOI: 10.2140/pjm.1979.80.293

Abstract

Let X be a compact subset of the complex plane. Let the module ℛ(X)𝒫_m be the space of all functions of the form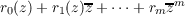

where each r_i is a rational function with poles off X. We prove that ℛ(X)𝒫₁ is dese in L^p(X) for all 1 ≦ p < ∞ and ℛ(X)𝒫₂ is dense in 𝒞(X) if X has no interior point. As corollaries, we also prove that ℛ(X)𝒫₂ is dense in lip (α,X) for all 0 < α < 1 and ℛ(X)𝒫₃ is dense in D¹(X) for the same X.

Mathematical Subject Classification 2000

Primary: 30E10

Milestones

Received: 17 May 1978

Revised: 10 August 1978

Published: 1 January 1979