On the topology of direct limits of ANRs

Richard Elam Heisey and Henryk Torunczyk

Vol. 93 (1981), No. 2, 307–312

DOI: 10.2140/pjm.1981.93.307

Abstract

Let {(X_n,a_n)} be a sequence of pointed, locally compact, finite-dimensional, nondegenerate, connected ANR’s. It is shown that the direct limit of the system

X1	→ X1 ×{a2}⊂ X1 × X2 → X1 × X2 ×{a3}⊂ X1 × X2 × X3
	→⋯

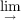is homeomorphic to an open subset of R^∞ = Rⁿ, R the reals. As a consequence, if f : X → Y is a homotopy equivalence between ANR’s as above then fⁿ : Xⁿ →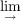Y ⁿ is homotopic to a homeomorphism.

Mathematical Subject Classification 2000

Primary: 57N20

Secondary: 54C55, 58B20

Milestones

Received: 15 June 1979

Revised: 7 February 1980

Published: 1 April 1981