|
|||||

|

|
|

|

|
|
Lie algebras and
growth in branch groups
Laurent Bartholdi |
|
Vol. 218 (2005), No. 2, 241–282
|
Abstract |
|
We compute the structure of
the Lie algebras associated to two examples of branch groups, and show that one has
finite width while the other, the Gupta–Sidki group, has unbounded width and Lie
algebra of Gelfand–Kirillov dimension log 3∕log(1 + We then draw a general result relating the growth of a branch group, of its Lie algebra, of its graded group ring, and of a natural homogeneous space we call parabolic space, namely the quotient of the group by the stabilizer of an infinite ray. The growth of the group is bounded from below by the growth of its graded group ring, which connects to the growth of the Lie algebra by a product-sum formula, and the growth of the parabolic space is bounded from below by the growth of the Lie algebra. Finally we use this information to explicitly describe the normal subgroups of G,
the Grigorchuk group. All normal subgroups are characteristic, and the number bn of
normal subgroups of G of index 2n is odd and satisfies limsupbn∕nlog 23 = 5log 23,
liminf bn∕nlog 23 = |
Keywords
Lie algebra, growth of groups, lower central series
|
Mathematical Subject Classification 2000
Primary: 20F14, 20F40, 17B70, 16P90, 20E08
|
Milestones
Received: 23 April 2002
Revised: 10 October 2003
Published: 1 February 2005
|
Authors |
| Laurent Bartholdi | |
| École Polytechnique Fédérale SB/IGAT/MAD, Bâtiment BCH CH-1015 Lausanne Switzerland |
|
| http://mad.epfl.ch/laurent/ | |
 |
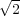
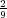 .
.